| |
| |
|
| ARCHIVE COPY - Abridged 2003 |
UP to TOP Down LIST DOWN |
 Musical Instruments - The steeldrums
Musical Instruments - The steeldrumsPresented by PANArt at the Conference on New Developments of the Steelpan
The Holiday Inn - La Villette, Paris, France - 20th of May 2000
 |
| ACOUSTICS |
Up TOP Up LIST DOWN |
MODES OF VIBRATION IN PLATES
In 1787 the German Physicist E. Chladni experimented with free vibrating plates. By sprinkling them with sand, he demonstrated that during the vibrations some areas did not vibrate. These areas are called nodal-lines. Their distribution determines the vibrational form (mode) in which an object is able to vibrate. Each mode has its own frequency. Objects vibrate simultaneously in various modes.
Sophie Germain (1776-1831), a French woman with autodidactical education, did important mathematical studies on vibrating plates. The term of curvature was very important in her studies on the Theory of Elasticity. Even Napoleon was fascinated with the phenomena and supported the research financially.
Commissioned by NASA, A. W. Leissa conducted many studies on plates and shells of different sizes and with varying boundary conditions. Many plates of a missile can vibrate!
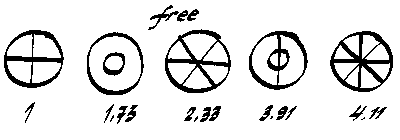
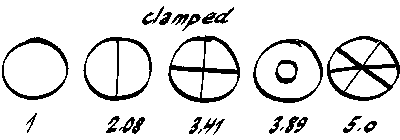
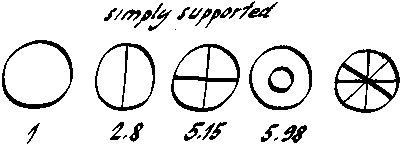
THE SKIRT
The sound spectrum of a skirt depends on the diameter, the thickness, E-modulus, length and the rim. The skirt influences the radiation of sound. The length should be a half wavelength of the lowest note. Reinforcement rings change the spectrum. The skirt is mainly an accumulator.
RECOUPLING
Holographic
interferometry makes it possible to visualise the vibrational mode patterns along with the
coupling effects. To produce these images, a note is excited electromagnetically
at a single frequency. At relatively low drive amplitudes, direct linear
coupling is observed between harmonically tuned sections and overtones of other
notes. Non-linear coupling between sections becomes evident as drive amplitudes
are increased.
SOUND SPECTRA
The harmonic overtones of a steelpan appear to have three different physical origins:
DEVELOPMENT OF PAN TUNING (The SOPRANO [or "Trinidad Tenor" pan])
| RELATED INSTRUMENTS |
Up LIST Up NEXT DOWN |
TABLA AND MRDANGA
These drums are tuned harmonically with an interesting technique. The centre of the drum is loaded with a special paste. The tuner tunes the rich sound spectrum by bringing modes together to almost the same frequency.
TIMPANI
The closed resonance body influences the frequency of the membrane. The loading lowers the modes of low frequencies and the stiffness of the air raises the frequencies of the axisymmetrical modes. The drum gets a correct pitch.
BELLS
Bells vibrate similar to plates. They don't have the three lowest modes which appear in a clamped shallow shell.
ZHONG BELLS
These 2000 years old Chinese bells have a two-frequency-system. Two strike points give two tones with nearly harmonically tuned overtones.
COWBELLS
These bells are called “Treichel” and are made of steel - 2mm thick, 2.5% carbon. Machine pressed forms are hammered under fire.
CHINESE OPERA GONGS
These gongs have a non-linear behaviour. The central section of the larger gong is nearly flat; it has a hardened spring behaviour, that means it glides downward in pitch. The smaller gong glides upward, it has a softening spring behaviour.
GONG AGENG
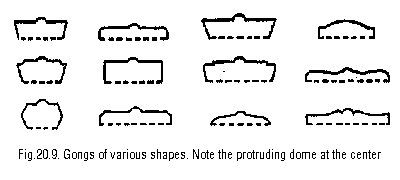
The large gong - gong ageng - has a clear pitch and a warm sound.
The double frequency system produces strong oscillations - onkas. The closer are the frequencies, the faster are the oscillations.
Ref: NH Fletcher and TD Rossing - The Physics of Musical Instruments
SINGING SAW
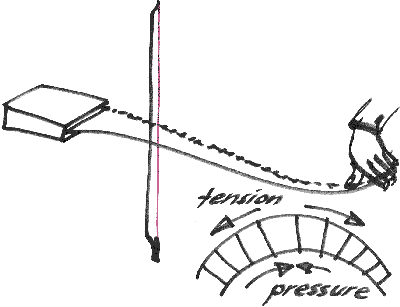
Pressure [compression] and tension, produced by the player at the ends of the saw, change the frequency.
CYMBALS
To get a rich crash the cymbals are hammered. The hammering increases the stiffness: the spectra is spread and the energy is shifted more to the overtones.
GAMELAN
The gamelan consists of metallophones, gongs, drums and a flute. Some of the metallophones build a couple, which is tuned ¾ tone different; they are played unisono and produce a music rich with oscillations.
| TECHNOLOGY |
Up LIST Up NEXT DOWN |
THE PROPERTIES OF METAL
STRESS + STRAIN DIAGRAM of a PANG-Sheet
SOUND AND MATERIAL
The important waves in a material for the sound [reproduction] are the bending-waves. Their velocity depends on the frequency (high frequency fast-wave) and on the bending-stiffness [of the material]. The thickness, the elastic modulus and the Poisson's constant (0.3 for steel) determine the bending-stiffness of the material. Curvature and edge conditions also influence the stiffness.
SOUND RADIATION
The radiation depends on the velocity of the bending-waves. The closer they are to the velocity of sound-waves in air, the better is the radiation. Higher frequencies radiate therefore better than lower frequencies. The speed of bending-waves in a tenor pan range from 68 to 200 meters per second. Although direct radiation from the note may be fairly weak, sound is radiated by the entire instrument, so that the radiated sound is fairly strong.
DAMPING
A vibrating system like a steelpan is damped by loss of energy through inner damping and transfer of energy into other vibrating areas. The different structure of the metal (strength-hardened, shocked, nitrided or age-hardened structure) and its composition, determines the inner damping. Also corrosion, colour-layers, coating and temperature influence the damping.

Musical Instruments - The steeldrums
Up LIST
Up NEXT
DOWN
The Modes of Vibration of a Steelpan Note and a Systematic Tuning Process
FELIX ROHNER - PANArt AG
THE PANArt Steelpan Manufacture participated in the establishment of about 60 steelbands and 30 school pan orchestras in the south-western part of Switzerland around the capital Berne. PANArt was founded by members of one of the oldest steelband from Switzerland, the Bernese Oil Company. The years 1991 and 92 were milestones in the history of this steelband. The Trinidad Casablanca Steel Orchestra came for a two weeks visit to our panyard; and the next year we were invited to stay in Belmont, [Port of Spain, Trinidad,] and to participate in the Trinidad and Tobago National PANORAMA steelband competitions. This experience at the birthplace of the steelpan evoked in us a great respect for the steelband artform; and on our return home, we could not hide this wonderful gift in the ground. There was much work to do.
I present you with a tuning process and terminology, which is the result of an intense collaboration with scientists and engineers; and of the study of instruments related to the steelpan together with the rich experiences we gained through our tuning projects. Due to an enormous boom [in the number] of steelbands [in Switzerland and Europe], we [felt obliged] to give 'the hammer*' and 'the knowledge*' into the hands of the steelbands. In the last two years [cf 2000], 40 steelbandsmen and steelbandswomen from Germany, France and Switzerland were trained in steelpan tuning.
The steelpan, a complex vibrating system: How does a pan note vibrate?
We decided to use the scientific designation, which has its origins in the beginning of the 19th century; when the German physicist Chladni experimented with vibrating plates.
We do an experiment:
Let us bring together 150 tenor pans of 150 tuners. We put sand on the lowest note. What do we see, when we excite it with a stick at the centre of the shell?
The sand is thrown to the boundaries: we see a mode of vibration, the one with the lowest frequency. It's the (0,0) mode, called the fundamental or tonic.
What do we see damping this mode with a finger in its centre, and exciting the shell on one side?
A pattern appears, a pattern of standing waves, divided with a nodal-line, which we see with the sand, because the metal does not move here. We see the (1,0) mode. [It is the octave.]
If we now make an impact, with a hard stick, on the curved side of the shell; we will see the third partial of the tone. [It will have a prominent nodal-line at 90º to the previously seen nodal-line of the octave; but is coloured] with a nodal-line in the octave direction. This is the (0,1) mode. Again, all notes will show this [additional] design of the next higher partial [the (1,1)]; but the frequency [of this (0,1) mode] will not be the same [on all the pans]. Some tuners will have tuned it to a third or a forth; others to a fifth or a fifth diminished.
The next two modes of vibration will be difficult to demonstrate [by methods of sand], because [the note is mechanically] too stiff.
Usually the lower one is the (2,0) mode. This is a very famous mode; bringing the brightness into the sound. This mode is a typical string-like mode, similar to the (1,0) and the (0,1) modes. A direct impact of energy into its amplitude, can provoke a strong attack character to the sound.
Its neighbour in frequency is the (1,1) mode (which we also call the twist mode). This mode vibrates like a bell and is often masked by the (2,0) mode. The (1,1) mode is not so strong in the sound spectra, because it is not [strongly] excited in its amplitude [by normal impacts central to the note].
Some of our 150 tenors will sound very bright, some have no 2nd octave.
The higher modes (2,1) and (1,2) are very difficult to manipulate by the tuner. Fortunately they are in good harmonic relationship between the 2nd and 3rd octaves. They are not very significant in the sound spectra, but they are quite important for the timbre, [especially] when you play fortissimo.
All pans show the same patterns. The 150 notes will have straight or curved nodal-lines, depending on the curvature of the shell and will show us the asymmetrical structure of an outer note of a steelpan.
I conclude: All objects have their manner to move, to vibrate, to transform an impact of energy into vibrations of the body. The steelpan note has its characteristic mode patterns, similar to a clamped plate with ratio 2:3 of length and width. For a steelpan tuner the first five modes of vibration are important. All tuner know, that the first three modes need to be tuned harmonically to produce a clear pitch and to play harmonically correct cords.
A Systematic Tuning Process
We all know the complexity of a tuning process. The study of other instruments like the gongs and gamelan, the physics of the string instruments and drums, lead to a tuning process which can be explained to our students. It is also the result of a dialogue between tuners. To understand our systematic tuning process, I need to give you the names of the areas of a steelpan note as we have defined them. These names have been proven through our daily tuning work.
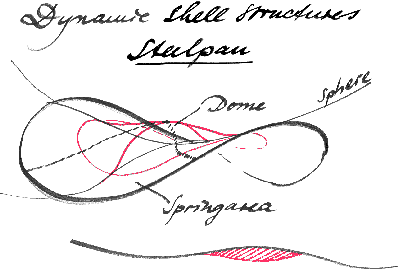
The geometry of a steelpan note may be described comprising 6 areas: the Heart, the Crown, the Trunk, the Roots, the Foundation and the Sphere.
The Heart
The heart is the most sensitive area. It is where the clamped plate or shell has a minimal bending-stiffness and the amplitude of is lowest mode, the fundamental. [It also coincides with being at that point, on the notes flexing surface, that moves to the maximum in amplitude.] Due to the unequal thickness of the outer notes of a steelpan, the heart is not in the centre of the note. It's right place is where the nodal-lines of the octave and the second overtone intersect.
The Crown
The crown is the area, where the low rise dome [graduates] into the spring area, the trunk. The crown is the base of the dome, which is the convex part of the vibrating system. Deformations of the crown influence extremely the fundamental mode.
The Trunk
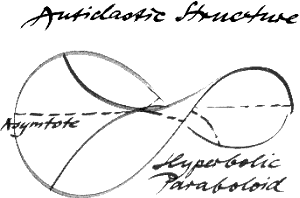
The trunk is a springlike structure. Its anticlastic form spread the rich number of vibrational modes of a clamped or simply supported shell.
The Roots
The roots area has a minimum in curvature. [At the base of the trunk,] it is the bridge to the double-curved stiff sphere [the outer limits of the note].
The Foundation
The foundation is a symmetric area of equal stiffness, to imagine like the asymptotes of an anticlastic structure.
Corresponding to these areas, we teach our students the heart-blows, crown-blows, trunk-blows, roots-blows and foundation-blow.
In case of chaos due to extreme external stress or bad geometry, we use the liberation-blow; a strong blow into the heart.
With this designation of the areas, we got an orientation; and it now becomes possible to tune a note by telephone. For example; if the fundamental frequency was to low, we could advice our customer, hundred miles away, to raise the heart by heartblows. The main problem with the steelpan is the weak construction of the fundamental.
The tuning process I'm going to explain, is a process which can be considered as a basic method to
construct a steelpan note; with the octave as a first overtone, together with the second overtone, in correct harmonic
relationship. It is used for tuning the middle and high range of the steelband instruments.
In some notes in the bass range there is a tuning with the fifth in the (1,0) mode and the second octave in the (0,1) mode.
For the tuning of notes in extreme position with large anticlastic form, the mode (0.1) is tuned to a double
octave. In that case, another method has to be used.
Our tuning process is caught in a pneumonic poem.
Each line describes a step in the tuning process.
To the sea!
Plant the tree!
Open the heart.
Move a stone.
Cathedral.
The first line, the first step - To the sea!
The first step demands the total destiffening of the roots and trunk area. This minimalisation process leads to an anticlastic structure. With heart-blows and foundation-blows from the back, the frequency of the fundamental mode will decrease to its minimum. At this point it will have its minimal curvature. If this is done, consequently the future note will have a stable structure. The tuner controls this step by listening for the decrease of the lowest frequency.
Second line, second step - Plant the tree!
The tree is the octave of the note. Upside root-blows stiffens the trunk, the frequency raises. This has to be done symmetrically, starting from the thicker roots, because thicker roots define more the sound through their stiffness. This is the most stable mode of the three lowest partials, it rarely goes out of tune.
Third line, third step - Open the heart!
In notes of the middle range, the fundamental will stay low during the second step, because its foundation is not touched. Sensitive heart-blows from the underside, now raises the fundamental until it reaches its absolute frequency. The dome is established and we have already a harmonically tuned tone. We arrived at the ratio 1:2. The octave will rarely change, because this manipulation happens on the nodal-line of the octave.
Fourth line, fourth step - Move a stone
To reinforce the structure, and to get another harmonic; roots-blows at the (0,1) mode, near the octave nodal-line, have to be made (we call them also support-blows). That way you move a stone to the foundation; that means you stabilise the foundation. This has to be done symmetrically.
Cathedral
To correct the ratios; we manipulate the crown from the upside, to lower the fundamental; and with upside trunk-blows, we lower the octave.
Here I have to stop and to ask: A brilliant one? Then you have to listen to the second octave - the (2,0) mode. The tuner analyses its frequency at the octave roots. It needs a flat note in octave direction to tune in the 2nd octave. But in many notes you have to lower the fifth to get the brightness.
 Do you want a mellow sound? Try to get in the fifth, perhaps you will loose the second octave and you will have a problem of balance. You see, at this moment, all depends on the character you want to give to the pan; or I should better say, the tuner is at a limit, where he has to set priorities: limits of material, limits of balance, limits of harmonic tuning etc.
Do you want a mellow sound? Try to get in the fifth, perhaps you will loose the second octave and you will have a problem of balance. You see, at this moment, all depends on the character you want to give to the pan; or I should better say, the tuner is at a limit, where he has to set priorities: limits of material, limits of balance, limits of harmonic tuning etc.
The tuning process I presented is based on the principals of symmetry, minimisation of stiffness, splitting of modes by anticlastic boundary. Tuning means a process which fulfills the following demands: Stability of the note, harmonic tone structure, control of recoupling.
One important aspect of the tuning has to be mentioned: The external tension. Each deformation provokes external tension in form of compression and tensile stresses in the microstructure as well as in the macrostructure of the shell. These stresses can be used for tuning, but it has to be said that external tension lead nearer to the yield point of the material, and the note will go out of tune faster, by hard playing or changes in temperature. There are methods to eliminate these tensions and to relax the structure; low tempering and blows into the centre of the note. The producers of springs use the same method; they overcharge the spring. It will be stronger afterwards.
Ladies and gentlemen, the pan is in development. It will be a voice in many regions of the world. The clamped shallow shell will get many forms if the tuners don't loose their vision of sound; and don't stop studying the laws of the metal.
Anywhere on the world a child is beating, at this moment, on a container. I'm sure, pan is not in danger.

Musical Instruments - The steeldrums
Up LIST
Up NEXT
DOWN
PANG - A Deepdrawn Form Leads to a New Shape - Dome Geometry -
To a New Sound and to High Stability
SABINA SCHÄRER - PANArt AG
FOR twenty five years steelpans have been made in Switzerland. The enormous demand for steelpans and problems with the materials available, provoked serious research.
PANArt Ltd invested in the development of the rawform to replace the [use of the bottom of the traditional 55 gallon steel] barrel. The initial idea was to create a form which the panmakers could use like a painter uses a canvas.
There are some tuners in Europe and the USA who have already worked on it. We have worked on this form for five years and it has opened to us new horizons. The rawform led to the dome geometry of the note, to a new tone quality and to new instruments.
The PANG instruments are all made of the same steel. Some of the instruments are made of the deepdrawn form I will introduce to you.
A lot of research was needed to find a solution to the following main questions:
How to get more durability? And how to get rid of the sinking by hand?
How to make the steel stronger?
Even specialists claimed that there is no way to harden sheet which has low content of carbon by tempering and quenching. By a stroke of good luck, we were told the story of the ancient Spanish smiths who shocked the tools in horse urine in order to harden them. This was the hint to the gas-nitriding process. Nowadays gas-nitriding is used for special tools and elements of machines and cars, which need a high surface hardness, fatigue strength and wear resistance. It is rare to find gas-nitriding in use with sheet metal, except in a few cases; but it can be found in use, in particular by the spring industries, as high tensile strength is needed for their springs. For durable instruments we need both, high tensile strength and fatigue strength.
What method could replace the strenuous sinking by hand?
The idea to have the sinking done by machines is not new. There have been attempts at machine made pans in England and in Trinidad. The industry uses various methods of metal forming: One of them is the deepdrawing. For the deepdrawing one uses soft steel, to be more precise, a steel which has a lot of elongation. But as we don't want to end up with soft pans, we split the forming and the hardening into two processes.
The automotive industry is faced with a similar problem: For forming they also need a steel with a lot of elongation, but in the end; the car body has to be rigid and buckle resistant and, at the same time, it should not be so hard as [] to break.
The Process to Make the Rawform
A blank of sheet metal is drawn over a tool by a press, then hardened and then attached to the skirt of stainless steel, which has a thickness of 1.25mm. The hardening process is based on nitrogen. In an oven at the temperature of 580 degrees Celsius, ammonia gas is split up on the glowing sheet surface into atomic nitrogen and atomic hydrogen. The nitrogen penetrates into the surface. The surface gets very hard; while the core stays softer. The duration of the hardening process determines the composition of the gradient composite material, the sandwich.
The Characteristics of the Rawform
The deepdrawn hemispherical form has a uniform thickness. The thickness all over is either 0.9mm or 1.1mm [dependant on which of the two forms manufactured is required at the time]. The combination of hard surface and pliable core unites several important qualities. These are the following:
The material is strong. The yield point and the tensile strength are almost twice as high as in common drum-head sheets. These properties are important for strong vibrating systems. Together with a good geometry stable sounds are the result. With 8 to 10 % of elongation, the material is pliable enough so that the shells can be formed. The properties of the material can be changed by heat treating and polishing. With heat treating the tensile strength increases about 10%. Even the elastic modulus also increases . With polishing away the hard surface, the sound can be changed. It is not necessary to chrome the PANG, because the gas-nitriding is a good corrosion protection and the skirt is made of stainless steel.
How we got to the new Dome Geometry
[With a view to maximise production and repeatability] it was difficult to make flat notes like [those] in the [traditional] steelpan. Bulging the very stiff hemisphere [of the instrument form] produced domes instead of flat notes. We first tried to flatten them until we realised that notes with a dome have a warmer sound. At that point we started exploring the dome geometry and then developed the present shape.
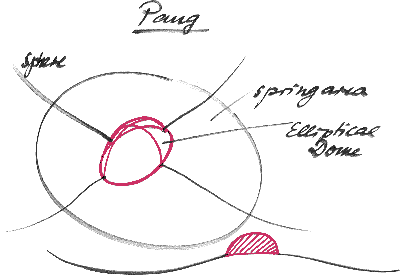
In the centre of the note an elliptical dome is first shaped with a hard stroke over a tool. Its dimension depends on the pitch of the note. Around the dome, a spring area is hammered. It connects the stiff dome with the stiff hemisphere. Stiffening rings around the dome help to reinforce the stability of larger springs.
 The Dome Geometry and the Tuning
The Dome Geometry and the Tuning
The dome is a stiff form in the centre of the note [the note-dome]. Due to its elliptical form, the (1,0) mode and the (0,1) mode are already spread. The higher modes are brought together and come to lie between the fifth above the second octave and the third octave. That means there is no second octave. The dome determines the ratios of the overtones to a great extent, because of the strong material, in which changes of shape provoke a spread of the overtones. Because the dome is very stiff, the boundary conditions need to be very soft. The dome is connected with the sphere [the outer boundary of the note] by a spring area. The spread between octave and fifth has been done already with the elliptical form of the dome, so the note gets nearly round. Therefore the fundamental has a symmetrical foundation which prevents distortions. The fundamental vibrates membranelike, due to its minimal curvature and stiff centre. One can compare it with the vibration of the stiff membrane of a loudspeaker.
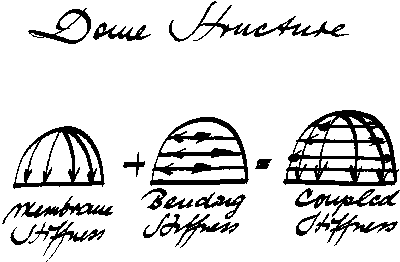
The properties of the Dome
In a plate only the bending-waves occur. In a shell other wave forms are possible. The impact on a dome provokes advantageous splitting in different wave forms. The dome couples the bending forces with the membrane forces, that means there is more resistance against tensile stress. Through an impact the shell will not come under tensile stress. That leads to stable sounds and a good intonation. The dome transforms the energy and leads it into the spring. The dome is flexible at its base and stiff in its centre. The position of different areas on the dome with equal stiffness determines the position of the foundation.
The New Sound
The note is struck at the dome. The energy flows first into the fundamental and then into the overtones. Due to the uniform thickness the nodal lines of the overtones go all through the dome (and are not struck in their amplitude). The octave and the fifth have approximately the same amplitude due to the round edge condition. The higher overtones are brought together and give a contribution to the timbre of the sound. So, the fundamental, the octave and the fifth produce a warm harmonic sound.
Other factors which influence the New Sound
The influence of the thickness and the elastic modulus on the velocity of the bending-wave is advantageous. The speed of bending-waves is closer to the speed of sound-waves in air, which allows a better sound radiation of the lower notes.
The skirt of stainless steel is a good accumulator and has good radiation.
The new instruments are altogether heavier than steelpans. A Ping [weighs] 7 kilos, a steelpan 3.5 kilos. They require a different way of playing.
| FOOTNOTE: * 'The hammer' and 'The knowledge' are cultural iconoclastic phrases in use on the West Indian islands of Trinidad and Tobago - the recognised birth place of the steeldrum instruments and in whose territories the steeldrums were declared in 1992 to be the National Instruments of the Republic - that embodies the meaning 'The ability to make and tune a steelpan'. The phrases carry a weighting of respect, coloured with a slight degree of awe, for anyone thus talented. They originate in the mid 1940's and became homogenised into the islands cultural consciousness by the early 1960's. The terms have been reinforced with use in the islands local cultural song-forms of Calypso and Soca. eEd |
| PANArt AG Engehaldenstr. 134 CH-3012 Bern Switzerland http://www.hang.ch info@hang.ch Tel: + 41 (0)31 301 3332 |
|
||||||

| [2nd Ed] © 2003: tobagojo@gmail.com - 20031026 - 1m20071228 - 2m20140615 Historic Update: 11 December 2003; Last Update: 29 June 2014 02:15:00 TT Processed by: JdeB - Islands Research |
UP |